The Trigonometric ratios of angle π/2 θ Thinking of θ as an acute angle (that ends in the 1st Quadrant), (π/2θ) or (90°θ) ends in the 2nd Quadrant where only sine of the angle is positive The (π/2θ) formulas are similar to the (π/2θ) formulas except only sine is positive because (π/2θ) ends in the 2nd Quadrant sin(π/2θ) = cosθCosθ1 sinθ = sinπ2 θ1 cosπ2 θ = 2sinπ4 θ2cosπ4 θ22 cos2π4 θ2 = sinπ4 θ2cosπ4 θ2 = tanπ4 θ2Cosθ =5/13 with π/2 < θ 96 results Pre Calculus Let θ be an angle in quadrant IV such that cosθ = 4/9 Find the exact values of csc θ and cot θ If cosθ cos^2 θ = 1, then sin^12 θ 3 sin^10 θ 3 sin^8 θ sin^6 θ 2 sin^4 θ 2 sin^2 θ – 2 =?

If Pi 2 Lt Theta Lt 3pi 2 Then Sqrt Tan 2 Theta Sin 2 Theta Is Equal To
Sin pi by 2 + theta is equal to
Sin pi by 2 + theta is equal to-\sin ^{2}\left(\cos ^{1}(1 / 2)\right)\cos ^{2}\left(\sin ^{1}(1 / 3)\right is equal to (a) \frac{13}{36} (b) \frac{59}{36} (c) \frac{5}{36} (d) none of thA) 3 5 B) 3 5 C) 4 3 D) 4 3 E) 4 5 the answers to ihomeworkhelperscom



1
Add sin^2(theta) to both sides Divide both sides by 2 Apply the square root to both sides Multiply top and bottom by sqrt(2) to rationalize the denominator Apply the arcsine, or inverse sine, to both sides Use the unit circle Note that sin(pi/4) = sqrt(2)/2 We were originally told that , and we just found Intersect those two intervals to getExample 116 involved finding the area inside one curve We can also use Area of a Region Bounded by a Polar Curve to find the area between two polar curves However, we often need to find the points of intersection of the curves and determine which function defines the outer curve or the inner curve between these two pointsSin is the sine function, which is one of the basic functions encountered in trigonometry It is defined for real numbers by letting be a radian angle measured counterclockwise from the axis along the circumference of the unit circle Sin x then gives the vertical coordinate of the arc endpoint The equivalent schoolbook definition of the sine of an angle in a right triangle is the
`sin^(2)sin^(2)70` is equal to _____ A 1 B 1 C 0 D 2 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queriesEvery sin, even the most minor sin, is egregious in God's sight, and enough to disqualify somebody from salvation That's why James says in James 210, that if you break one of the points of the Law, you're guilty of the whole thing But James is not saying that all sins are equally egregious, which was the application the professor wasList of trigonometric identities In mathematics, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined Geometrically, these are identities involving certain functions of
The inverse sin of 1, ie sin1 (1) is a very special value for the inverse sine functionRemember that sin1 (x) will give you the angle whose sine is x Therefore, sin1 (1) = the angle whose sine is 1 The Value of the Inverse Sin of 1 As you can see below, the inverse sin1 (1) is 90° or, in radian measure, Π/2 '1' represents the maximum value of the sine function It happens at Π/2Let's start with the left side since it has more going on Using basic trig identities, we know tan (θ) can be converted to sin (θ)/ cos (θ), which makes everything sines and cosines 1 − c o s ( 2 θ) = ( s i n ( θ) c o s ( θ) ) s i n ( 2 θ) Distribute the right side of the equation 1 − c o s ( 2 θ) = 2 s i n 2 ( θ)Sin^ {2} (theta) cos^ {2} (theta) =1 The Pythagorean trigonometric identity, also called simply the Pythagorean identity, is an identity expressing the Pythagorean theorem in terms of trigonometric functions Along with the sumofangles formulae, it is one of the basic relations between the sine and cosine functions The identity is




Cos 2pi Theta Cosec 2 Pi Theta Tan Pi 2 Theta Sec Pi 2 Theta Cos Theta Cot Pi Brainly In
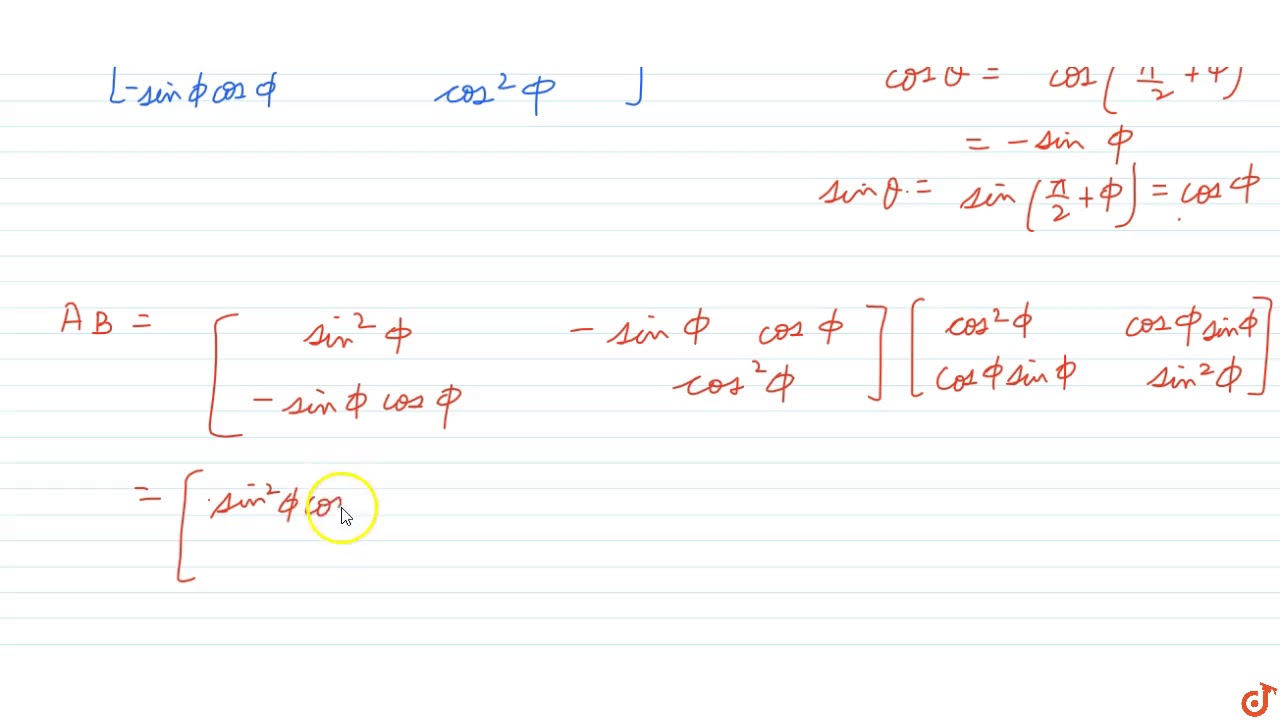



If Theta Phi Pi 2 Then Show That Cos 2theta Costhetasintheta Costhetasintheta Sin 2thet Youtube
Given that sin−1(1−x)−2sin−1x = 2π let x = siny ∴ sin−1(1−siny)−2y = 2π ⇒ sin−1(1−siny) = 2π 2y ⇒ 1−siny = sin(2πMath 109 T6Exact Values of sinθ, cosθ, and tanθ Review Page 2 61 By memory, complete the following table θ 0 π 6 π 4 π 3 π 2 2π 3 3π 4 5π 6 π 3πNow, write the values of sine degrees in reverse order to get the values of cosine for the same angles As we know, tan is the ratio of sin and cos, such as tan θ = sin θ/cos θ Thus, we can get the values of tan ratio for the specific angles Sin Values sin 0° = √(0/4) = 0 sin 30° = √(1/4) = ½ sin 45° = √(2/4) = 1/√2




Sin 2 Pi 6 Cos 2 Pi 3 Tan 2 Pi 4 1 2 Youtube




Basic Trigonometric Functions Trigonometry Socratic
Transcript Ex 22, Find the values of sin (π/3 −"sin−1" (−1/2)) is equal to(A) 1/2 (B) 1/3 1/4(D) 1Solving sin1 ((−𝟏)/𝟐)Let y = sin1 ((−1)/2) y = − sin1 (1/2) y = − 𝛑/𝟔 We know that sin−1 (−x) = − sin −1 x Since sin 𝜋/6 = 1/2 𝜋/6 = sin−1 (𝟏/𝟐)Thus,sin−1 (−1/2) = (−π)/6SolvingYes, using the trigger, an entity sine squared is equal to one minus coastline to David about it too We can rewrite this integral as 1/8 hands the integral of one minus co sign too, ext Yes, and this is just equal to 1/8 times x minus Sign to x divided by two plus C or our islandsA cosθ – sin θ B sinθ – cosθ C sinθ cosθ D cotθ – tanθ
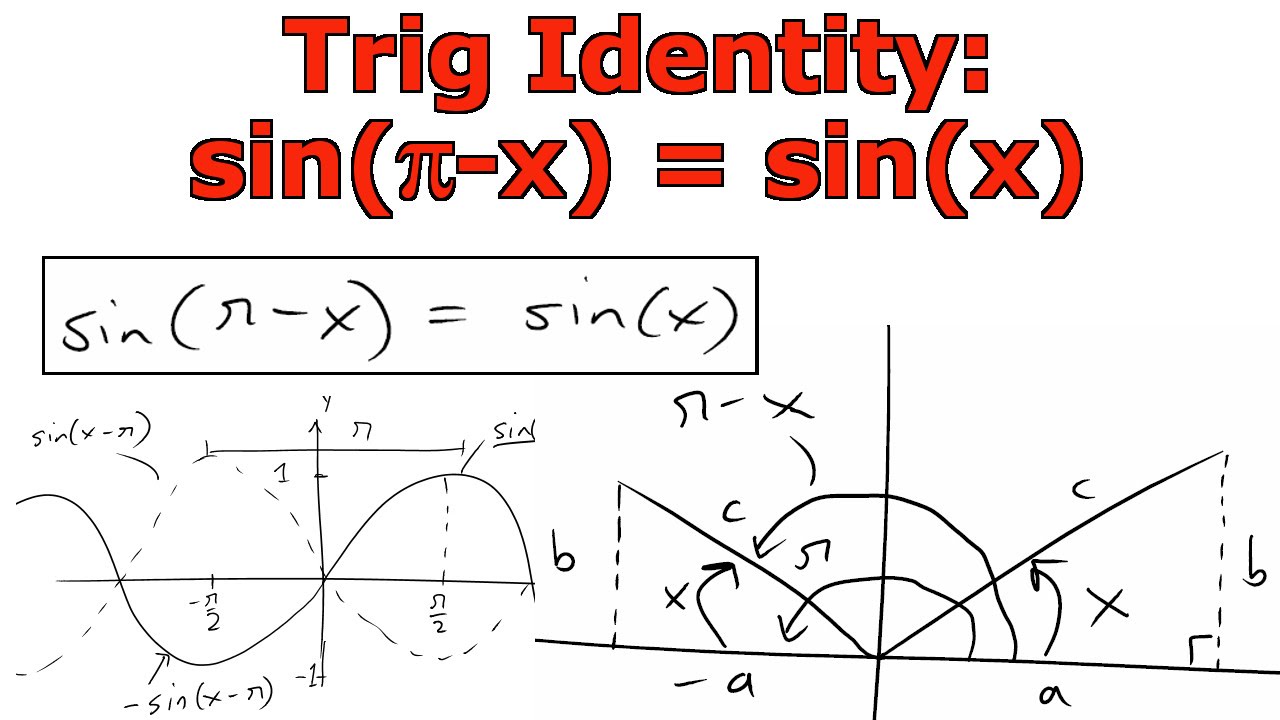



Trigonometric Identity Sin P X Sin X Youtube



1
The usual convention is that sin2(X) = (sin(X))2 So for your example 1 / 4 is correct sin(30 ∘) = 1 / 2 , thus sin2(30 ∘) = (sin(30 ∘))2 = (1 / 2)2 = 1 / 4 However, sin(900 ∘) = sin(180 ∘ ⋅ 5) = sin(180 ∘) = 0 because sin(180 ∘ ⋅ k) = 0 for any integer kWhat we're going to do in this video is prove that the limit as theta approaches zero of sine of theta over theta is equal to one so let's start with a little bit of a geometric or trigonometric construction that I have here so this white circle this is a unit circle let me label it as such so it has radius one unit circle so what does the length of this salmoncolored line represent well theSimplify = a 2 − a 2 sin 2 θ Factor out a 2 = a 2 (1 − sin 2 θ) Substitute 1 − sin 2 x = cos 2 x = a 2 cos 2 θ Take the square root = a cos θ = a cos θ a 2 − x 2 = a 2 − (a sin θ) 2 Let x = a sin θ where − π 2 ≤ θ ≤ π 2 Simplify = a 2 − a 2 sin 2 θ Factor out a 2 = a 2 (1 − sin 2 θ) Substitute 1 − sin 2 x = cos 2 x = a 2 cos 2 θ Take the square root = a cos θ = a cos θ



Pplato Flap Math 1 6 Trigonometric Functions




Sin 7theta Sin 4theta Sintheta 0 0 Theta Pi 2
How to solve trig equations, sin (θ)=1/2, θ in 0, 360)Sin(x) lim = 1 x→0 x In order to compute specific formulas for the derivatives of sin(x) and cos(x), we needed to understand the behavior of sin(x)/x near x = 0 (property B) In his lecture, Professor Jerison uses the definition of sin(θ) as the ycoordinate of a point on the unit circle to prove that lim θ→0(sin(θ)/θ) = 1Tan (theta) = oppo/adj = 2/3 assume oppo = 2 and adj = 3 (you can assume them to be anything proportional to 2 and 3 respectively) sin (theta) = oppo/hypo we know that oppo = 2 by pythagoras theorem hypo = sqrt (oppo^2adj^2) therefore,hypo = sqrt (49) = therefore sin (theta) = 2/ = 055




If Pi 2 Lt Theta Lt 3pi 2 Then Sqrt Tan 2 Theta Sin 2 Theta Is Equal To
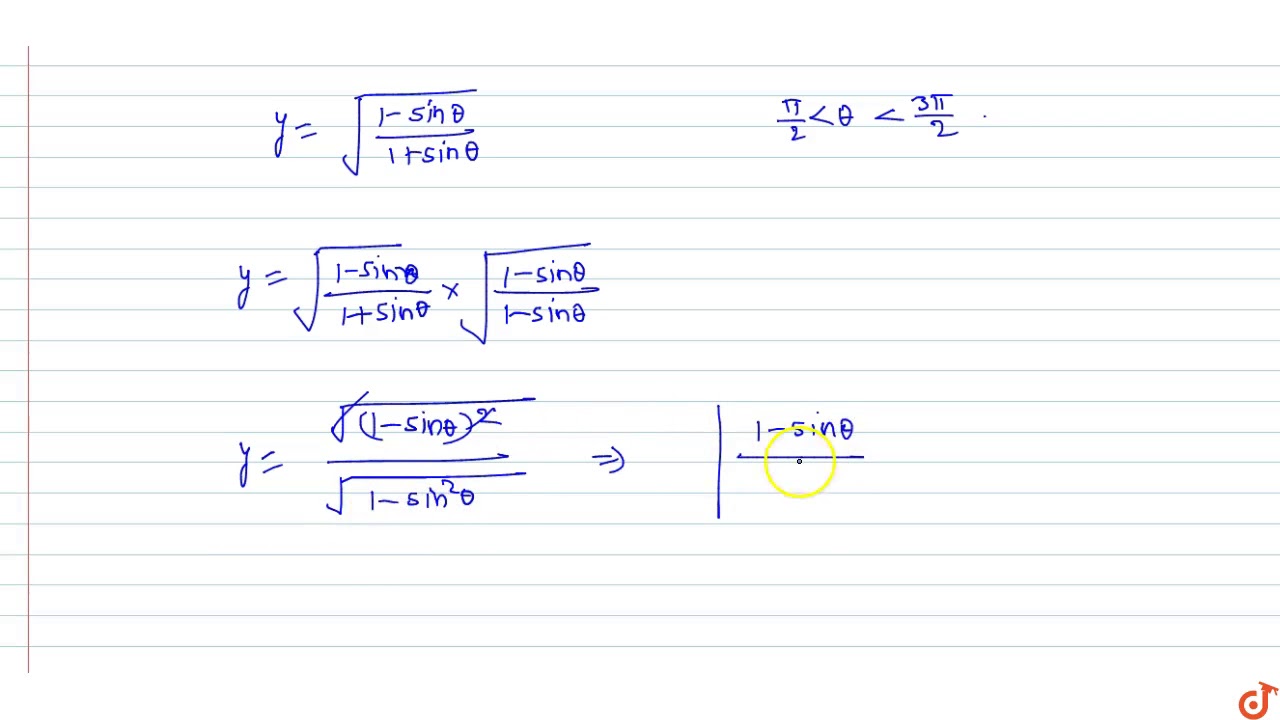



If Pi 2 Lt Theta Lt 3pi 2 Then Sqrt 1 Sin Theta 1 Sin Theta Is Equal To Youtube



0 件のコメント:
コメントを投稿